 Ellipses and Ellipsoids
Ellipses and Ellipsoids Ellipses and Ellipsoids
Ellipses and Ellipsoids

|

If putting the ellipse into a rectangle looks too tough, you can just measure how long it is to get A, and then measure how fat it is to get B, then multiply the two results together. You then divide by 4 and multiply by pi.
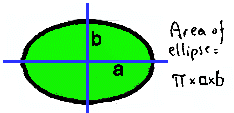
Sometimes you're given the lengths of the "longest radius" (shown here as a) and the "shortest radius" (shown here as b). Obviously in our diagrams a = ½A and b = ½B in which case you get this formula:
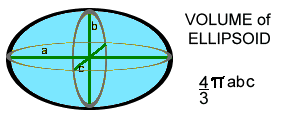 In the same way that a circle turns into a solid sphere, an ellipse can become
a solid "ellipsoid".
In the same way that a circle turns into a solid sphere, an ellipse can become
a solid "ellipsoid".
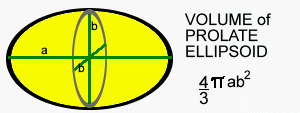 Suppose you get a sphere and stretch it to make a longer and thinner shape
(a bit like a rugby ball or a melon) this is called a PROLATE ELLIPSOID.
If you chop it in half to get a circle, then the volume is the area of the circle
times by 2/3rds of the major axis. (The major axis is the maximum length from one end to another.)
Suppose you get a sphere and stretch it to make a longer and thinner shape
(a bit like a rugby ball or a melon) this is called a PROLATE ELLIPSOID.
If you chop it in half to get a circle, then the volume is the area of the circle
times by 2/3rds of the major axis. (The major axis is the maximum length from one end to another.)
 However if you get a sphere and squash it to make a shorter fatter shape
(a bit like a SMARTIE or a burger) this is called an OBLATE ELLIPSOID.
If you chopped it through the middle to get a circle, then the volume is
the area of the circle times by 2/3rds of the minor axis. (If your "burger" is lying flat on a table, the minor axis is the height.)
However if you get a sphere and squash it to make a shorter fatter shape
(a bit like a SMARTIE or a burger) this is called an OBLATE ELLIPSOID.
If you chopped it through the middle to get a circle, then the volume is
the area of the circle times by 2/3rds of the minor axis. (If your "burger" is lying flat on a table, the minor axis is the height.)
Many thanks to "Pete" who contacted us with corrections to this page in April 2005.
