The (Amazing) Alternate Segment Theorem!

In Savage Shapes there's a chapter on some of the strange things that happen with circles - but sadly there wasn't room to put everything. A Murderous Maths fan called ISLA KENNEDY asked us about "The Alternate Segment Theorem" so here it is...

• Here's a circle with a tangent PQ drawn touching it:
• The chord CD is drawn from the place the tangent touches up to somewhere on the circle
• Point Z is marked anywhere on the circle, and is joined to the ends of the chord with two lines.

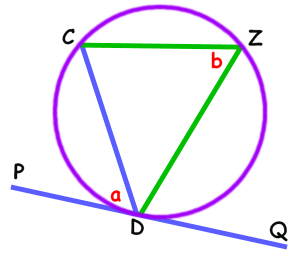
Here's the funny bit:

The angle between the chord and the tangent is ALWAYS equal to the angle "in the alternate segment".

In other words, in our diagram, angle a is always the same as angle b.

If you're not sure what segments or chords are look at our guide to Names of Bits of Circles

 

If you've read Savage Shapes, you'll know that you're not allowed just to say something without explaining why it always works, so here's the proof. (You might want your copy of Savage Shapes handy to check on some of the details we're about to use.)

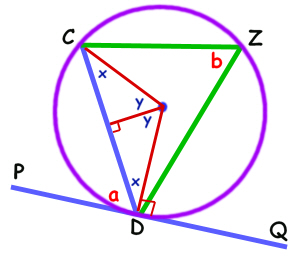We've drawn a couple of radii (i.e. lines from the centre of the circle, we've made them red for clarity) to the ends of the chord. This makes an isosceles triangle with two equal angles which we've marked "x". (See page 104 of Savage Shapes.)

We split the isosceles triangle into two identical little right angled triangles. Each of them has an angle y at the centre of the circle.

The angle between the radius and the tangent is 90º (see page 114).

Now we just play with the angles a bit:

• As the angle between a tangent and a radius is 90º then angles a + x = 90.
• As the two small angles in a right angled triangle add up to 90º
  then y + x =90.
• If a + x =90 and y + x = 90 , therefore a = y.
• We need to use the theorem "The angle at the centre is twice the angle at the circumference" (see page 111). The angle at the centre is 2y, so the angle b at the circumference = y.
• If b = y and a = y , therefore a = b. We've done it!

 

You can go on to show that this theorem works for the angles over 90º too!

In this case, now we know that a = b we can show that s = t.

• As the line PQ is straight, we can see that a + s = 180.
• As angles b and t are opposite angles in a cyclic quadrilateral, we know that b + t = 180. (See page 104).
• As we've proved that a = b we can put a + s =180 and a + t =180.
  Therefore s = t.

This is clever stuff - so if you've understood it WELL DONE!

Back to Savage Shapes

Murderous Maths Main Index Page